混合励磁型多自由度球形电机的偏转分析

doi:10.12141/j.issn.1000-565X.220610

摘要/Abstract

摘要：

为解决现有多自由度电机的偏转运动部分存在转矩不足、转矩波动大和运动范围小的问题，提出了一种混合励磁型多自由度球形电机。在介绍该电机的基本结构和运行原理的基础上，提出了基于多节点许-克变换的磁场解析法，以解决该电机使用三维有限元法进行磁场计算时速度慢的问题。该方法利用离散的线电流对电机的永磁体和绕组进行等效，并将复杂的气隙区域分别通过多节点许-克变换和指数变换转化为圆环区域，利用圆环区域中磁标量位的解析解计算单根线电流区域中的磁场信息，并通过叠加计算整体的磁场信息。而后利用各区域坐标点的转化关系计算原气隙区域的磁场信息，计算获得径向磁密之后通过安培定律计算偏转部分的电磁转矩。本研究采用解析法和三维有限元法建模分析了电机的磁场特性和偏转转矩特性，两种方法的磁场计算结果的吻合度较高，解析法相对于有限元法在幅值上的最大误差分别为7.3%和3.92%，验证了解析法的准确性。为了进一步验证，研制了样机并搭建了实验平台，进行了电机静偏转转矩的测试，结果表明，该电机具有较高的偏转转矩，最大偏转转矩为2.13 N·m，且实验值和两种理论的转矩计算结果的误差较小，实验结果与有限元法、解析法的计算结果误差分别为22.62%和20.13%，验证了解析法建模的有效性。

关键词: 混合励磁, 多自由度, 许-克变换, 磁场, 转矩

Abstract:

In order to solve the problems of small torque, large torque fluctuation and small motion range in the deflection motion part of the existing multi-degree-of-freedom motor, a hybrid excitation multi-degree of freedom spherical motor was proposed. After introducing the basic structure and operation principle of the motor, this paper proposed a magnetic field analysis method based on multi-node Schwarz-Christoffel (S-C) transform to solve the problem of slow speed in the magnetic field calculation of the motor when using the three-dimensional (3D) finite element method. This method uses discrete line currents to be equivalent to the permanent magnets and windings of the motor, and converts the complex air gap region into the torus region through multi-node S-C transform and exponential transform, respectively. And it uses the analytical solution of the scalar position in the torus region to calculate the magnetic field information in the current region of a single line, and calculates the overall magnetic field information by superposition. Then, the magnetic field information of the original air gap region was calculated by using the conversion relationship of the coordinate points in each region. After calculating the radial magnetic density, the electromagnetic torque of the deflected part was calculated by Ampere’s law. In addition, the analytical method and the three-dimensional finite element method were used to model and analyze the magnetic field characteristics and deflection torque characteristics of the motor. Through comparison, it is found that the magnetic field calculation results of the two methods are in good agreement, the maximum errors in amplitude of the analytical method relative to the finite element method are 7.3% and 3.92%, respectively,which verifies the accuracy of the analytical method. In order to further verify its validity, a prototype and an experimental platform were developed to test the static deflection torque of the motor. The results show that the motor has a high deflection torque, and the maximum deflection torque is 2.13 N·m. The errors between the experimental results and the calculation results of the finite element method and the analytical method are 22.62% and 20.13%, respectively, which verifies the effectiveness of the analytical method modeling.

Key words: hybrid excitation, multi-degree of freedom, Schwarz-Christoffel transform, magnetic field, torque

中图分类号:

TM301

曹江华, 曾炳森, 杨向宇, 等. 混合励磁型多自由度球形电机的偏转分析[J]. 华南理工大学学报(自然科学版), 2023, 51(12): 95-106.

CAO Jianghua, ZENG Bingsen, YANG Xiangyu, et al. Deflection Magnetic Field Analysis and Deflection Torque Calculation of a Hybrid Excitation Multi-Degree of Freedom Spherical Motor[J]. Journal of South China University of Technology(Natural Science Edition), 2023, 51(12): 95-106.

图/表 20

图1

表1

图2

图3

图4

图5

图6

图7

图8

图9

图10

图11

图12

图13

图14

图15

表2

近年所提出的多自由度电机的部分参数"

类型	定子外直径/mm	最大偏转角度/（°）	$T s m a x$ / （N·m）	$M 70 %$ /%	$M 50 %$ /%
磁阻型^［9］	140	±28.5	1.05	26	40
分段轮毂^［23］	250	±11.5	11.2	52	74
圆柱台阶式^［7］	90	±27	0.31	39	69
HE-MDOFSM	166	±29	2.13	99	100

表2

图16

图17

图18

参考文献 23

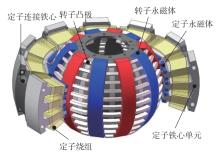
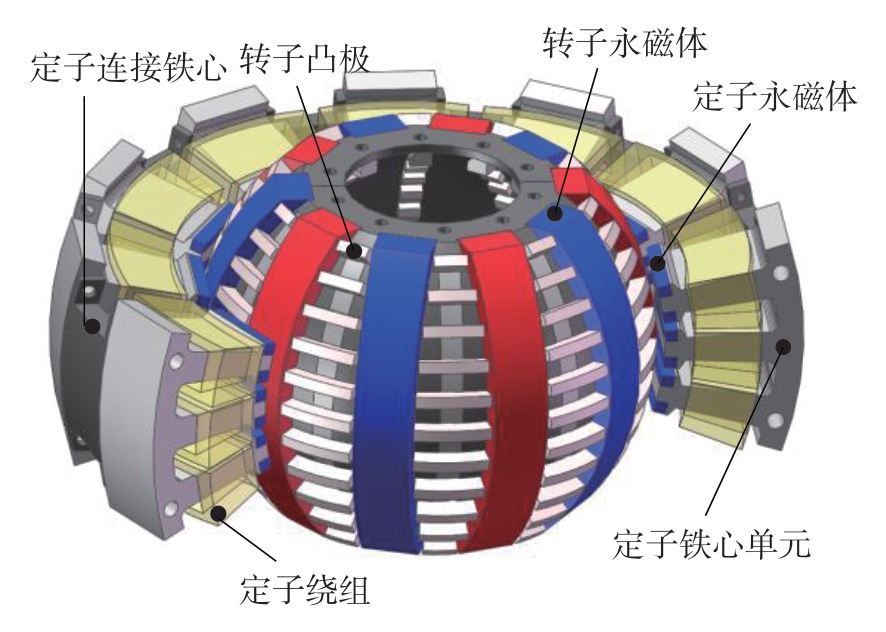
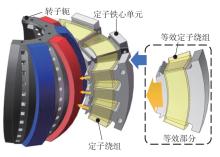
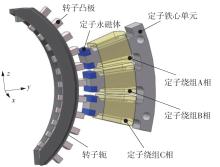
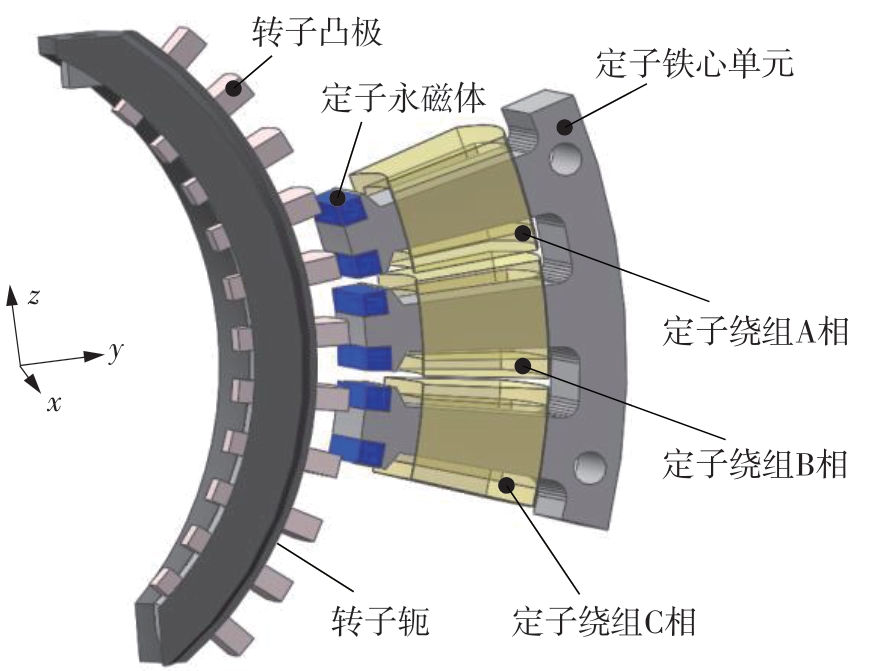
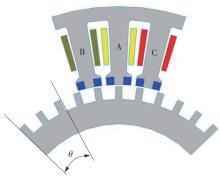
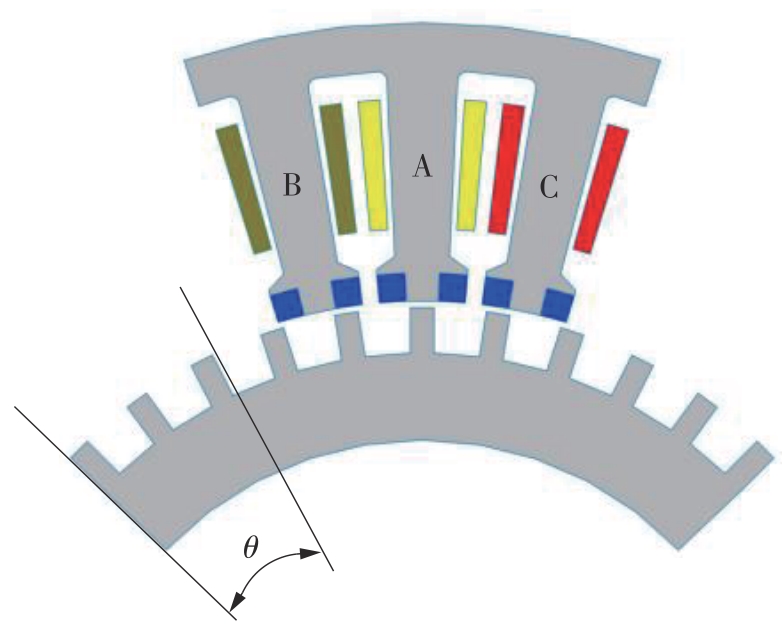
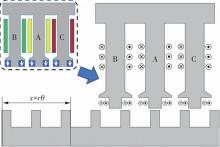
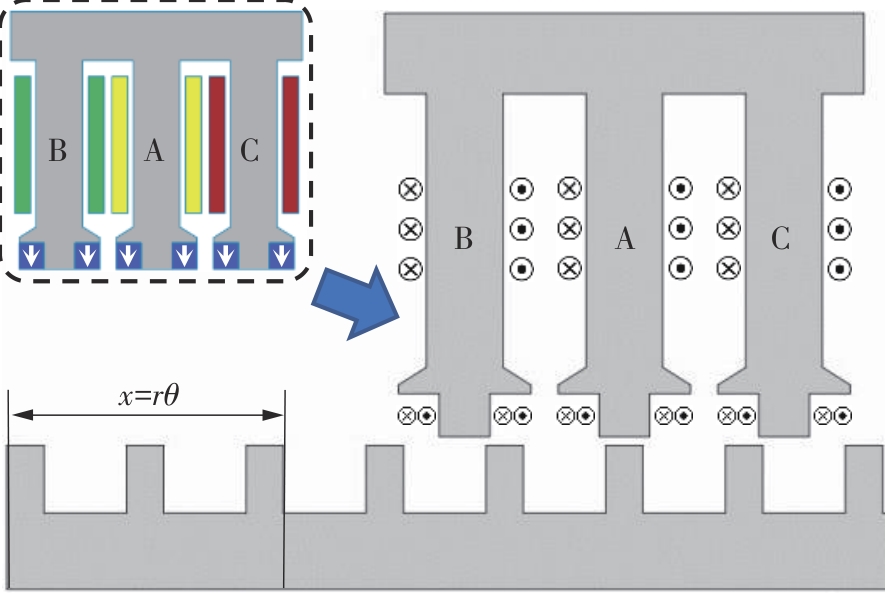
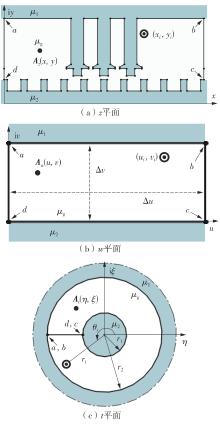
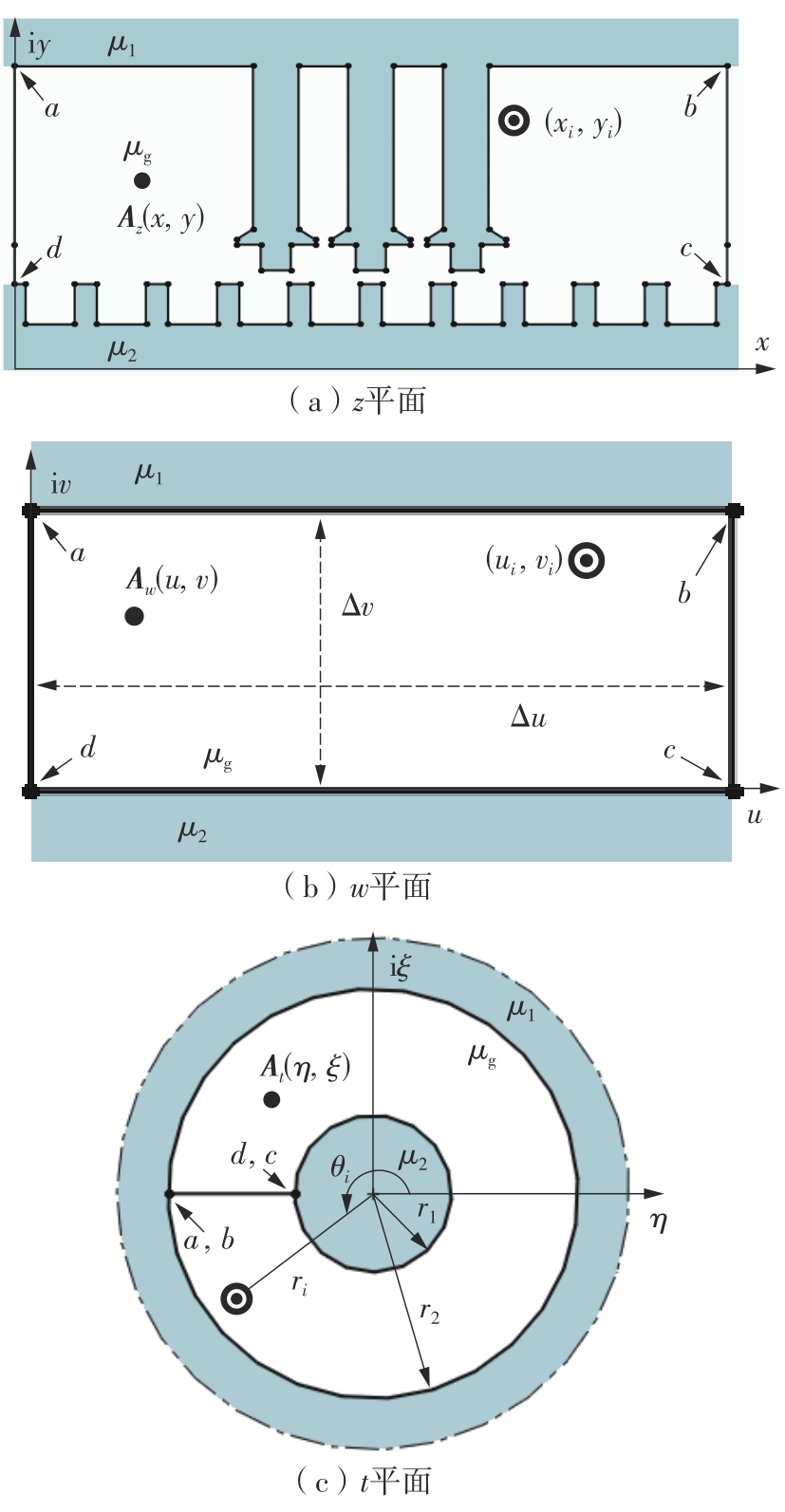
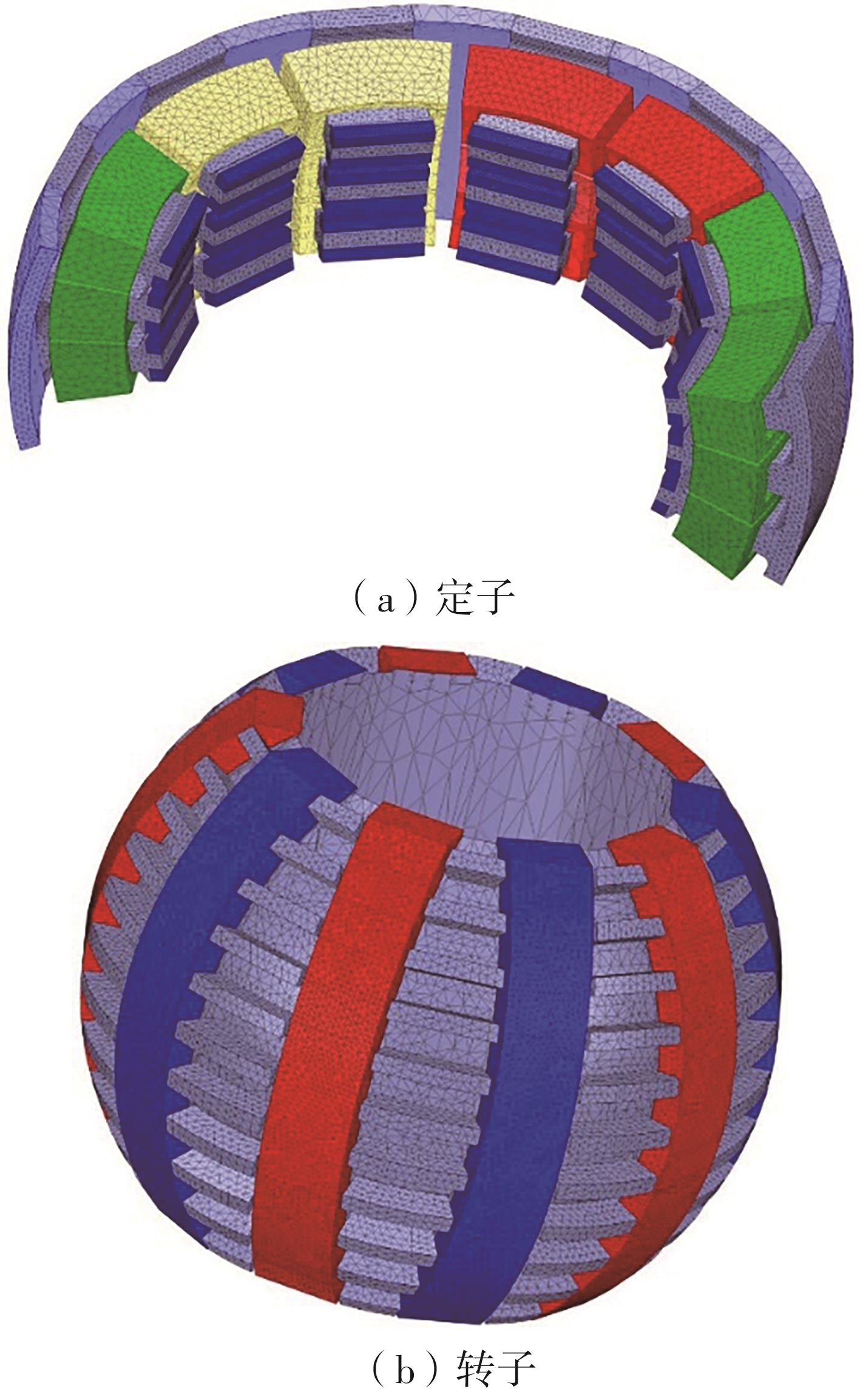
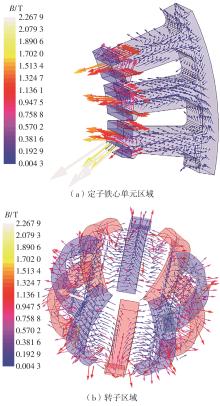
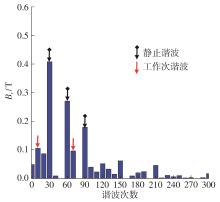
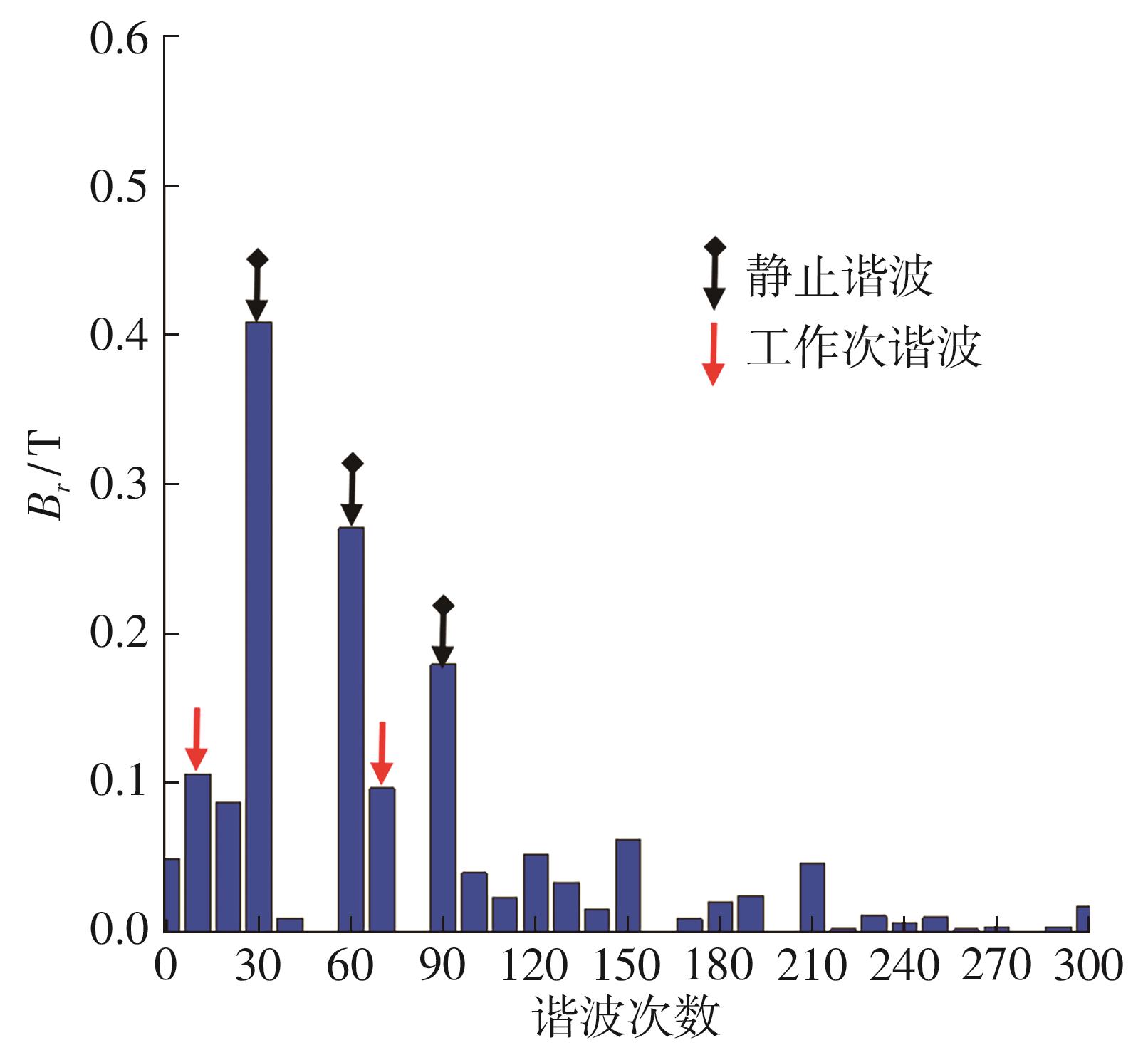
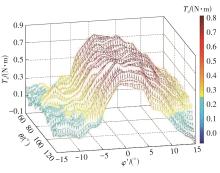
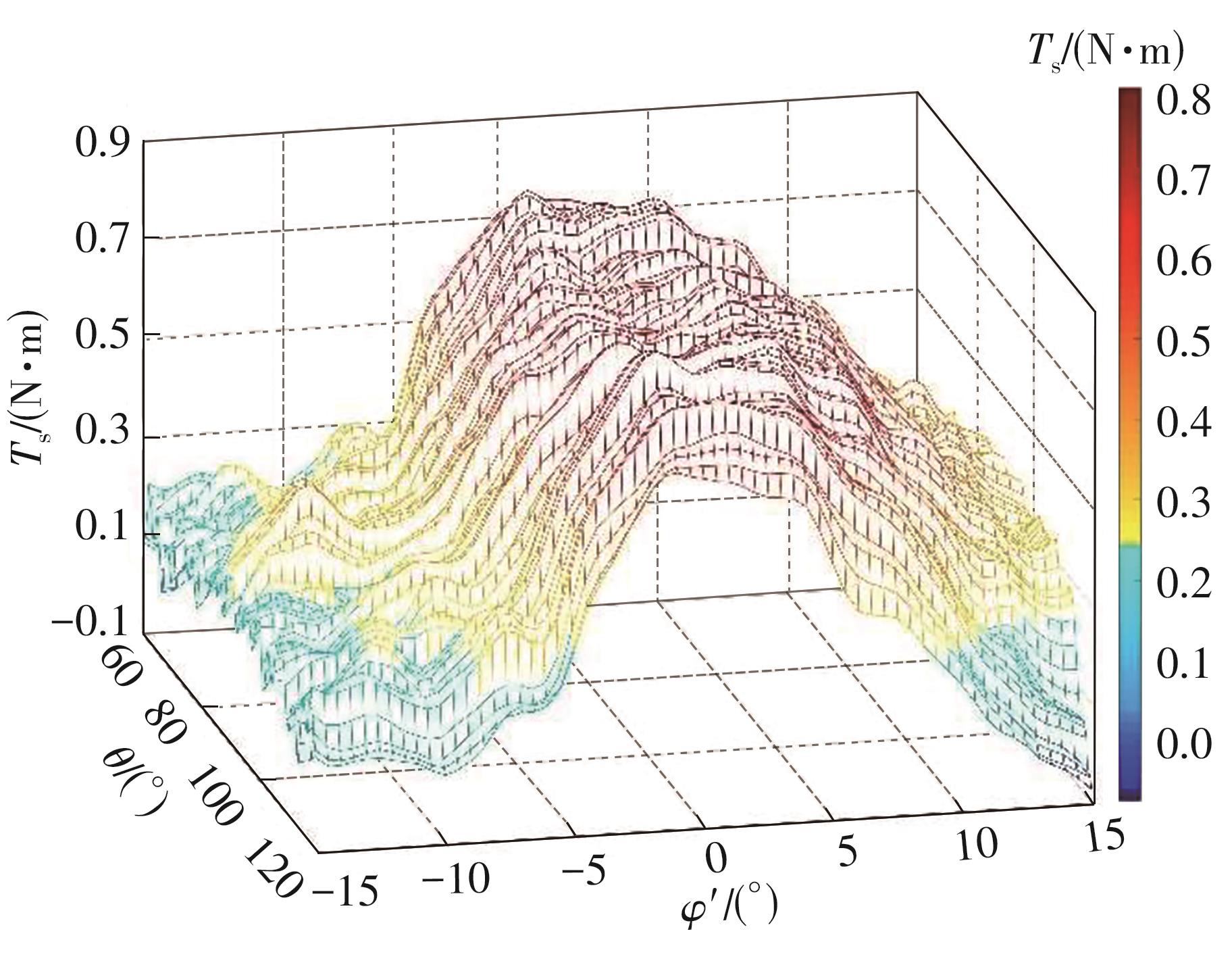
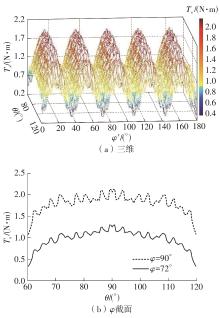
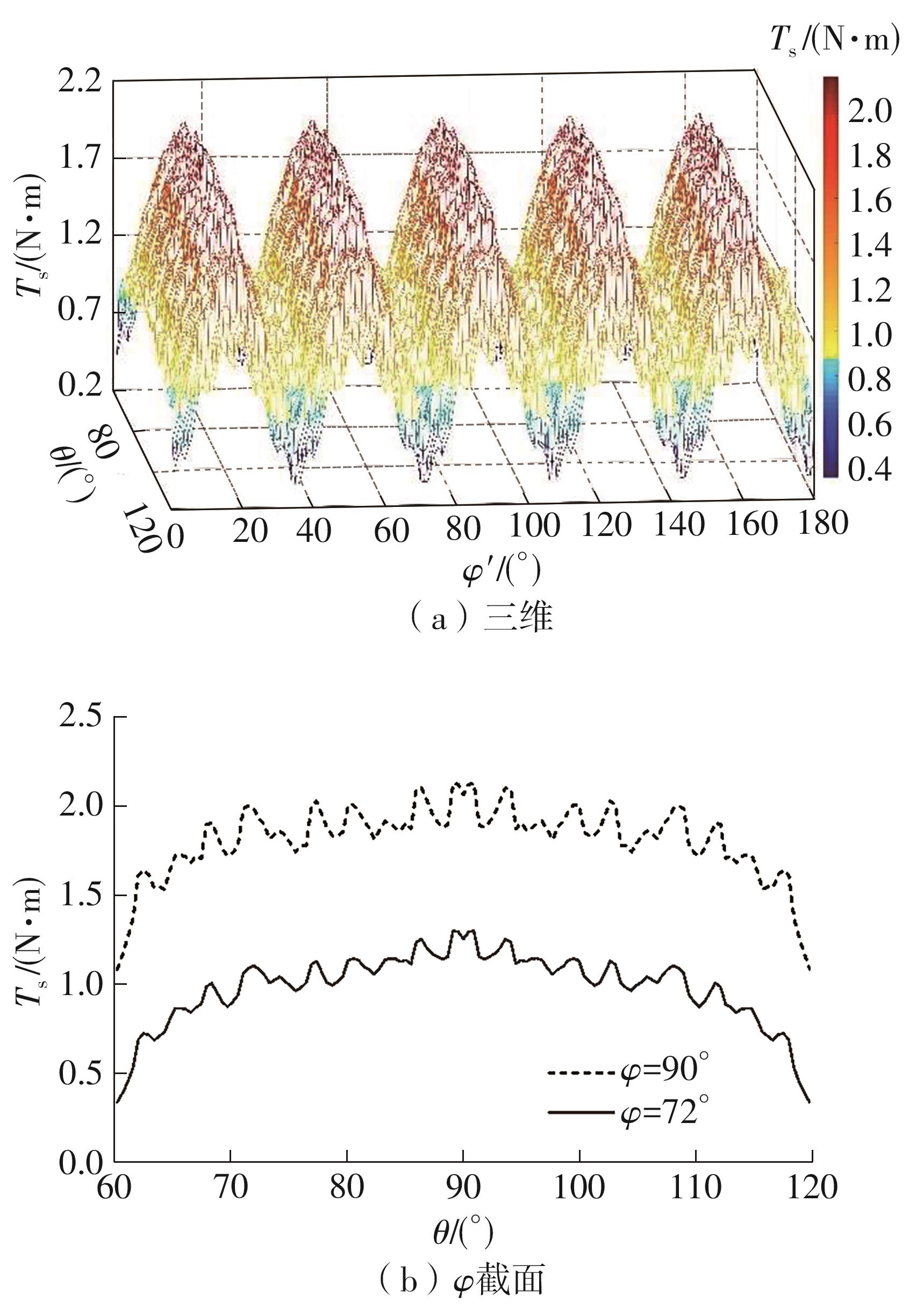
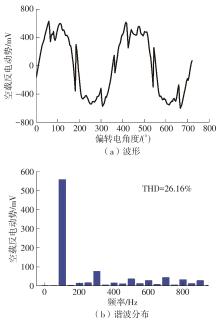
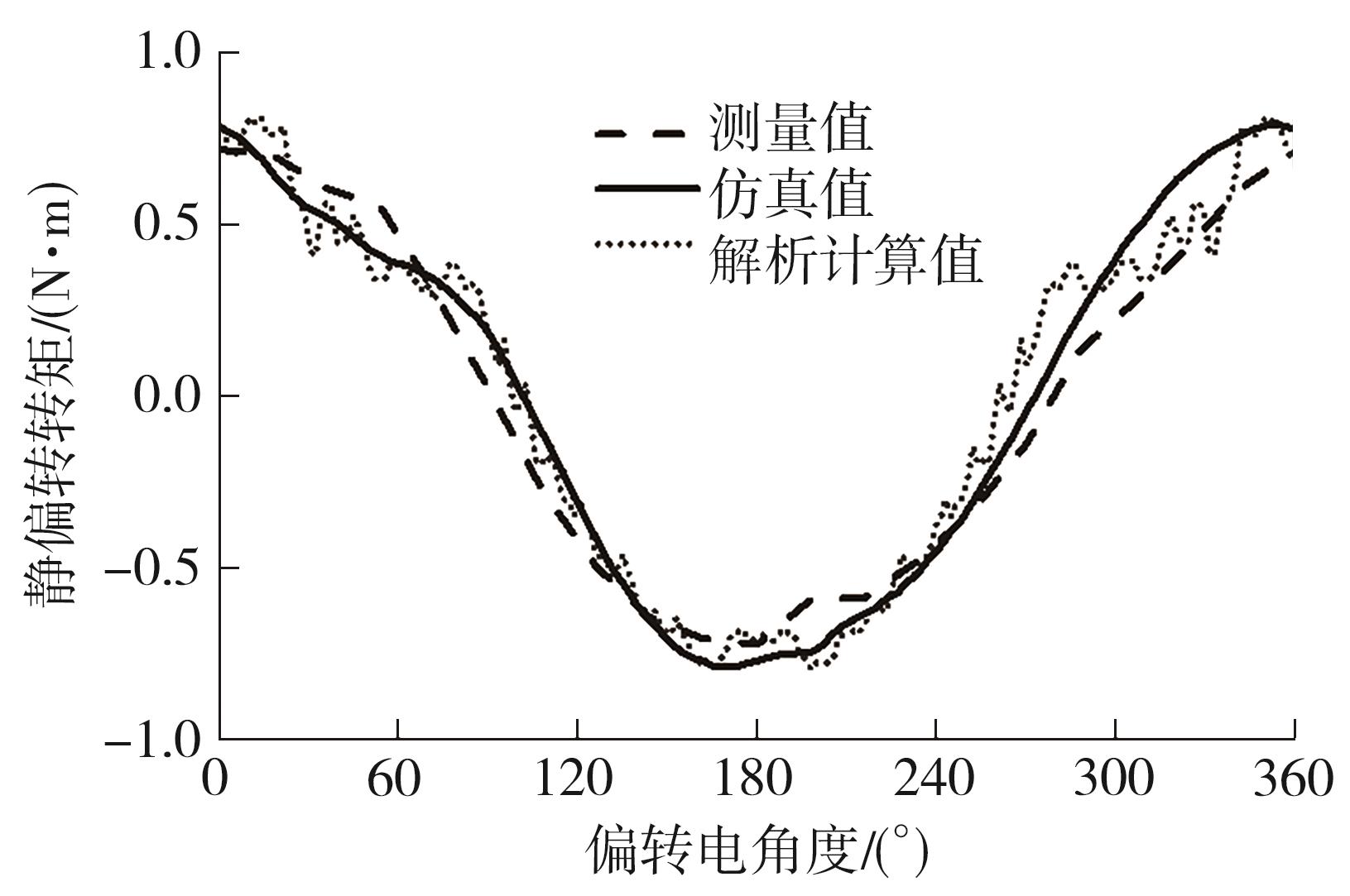
1	FERNANDES J F P， BRANCO P J C，et al ．The shell-like spherical induction motor for low-speed traction：electromagnetic design，analysis，and experimental tests［J］．IEEE Transactions on Industrial Electronics，2016，63（7）：4325-4335.
2	KASASHIMA N， ASHIDA K， YANO T，et al ．Torque control method of an electromagnetic spherical motor using torque map［J］．IEEE/ASME Transactions on Mechatronics，2016，21（4）：2050-2060.
3	LI H F， LI T M ．End-effect magnetic field analysis of the Halbach array permanent magnet spherical motor［J］．IEEE Transactions on Magnetics，2018，54（4）：1-9.
4	李斌，张硕，李桂丹，等．基于球谐函数的动量球定子磁场分析［J］．电工技术学报，2018，33（23）：5442-5448.
	LI Bin， ZHANG Shuo， LI Guidan，et al ．Stator magnetic field analysis of reaction sphere based on spherical harmonics［J］．Transactions of China Electrotechnical Society，2018，33（23）：5442-5448.
5	YAN L， CHEN I M， LIM C K，et al ．Design and analysis of a permanent magnet spherical actuator［J］．IEEE/ASME Transactions on Mechatronics，2008，13（2）：239-248.
6	BAI K， XU R Y， LEE K M，et al ．Design and development of a spherical motor for conformal printing of curved electronics［J］．IEEE Transactions on Industrial Electronics，2018，65（11）：9190-9200.
7	HE J X， LI G L， ZHOU R，et al ．Optimization of permanent-magnet spherical motor based on Taguchi method［J］．IEEE Transactions on Magnetics，2020，56（2）：1-7.
8	李国丽，孙涛，周睿，等．基于转矩模型和改进MOPSO的永磁球形电机磁极优化［J］．电机与控制学报，2022，26（6）：112-120，133.
	LI Guoli， SUN Tao， ZHOU Rui，et al ．Poles optimization of permanent magnet spherical motors based on torque model and improved MAPSO［J］．Electric Machines and Control，2022，26（6）：112-120，133.
9	王群京，乔元忠，鞠鲁峰，等．一种凸极式磁阻型球形电机的结构设计［J］．电机与控制学报，2021，25（1）：90-102.
	WANG Qunjing， QIAO Yuanzhong， JU Lufeng，et al ．Structural design of a salient pole type reluctance spherical motor［J］．Electric Machines and Control，2021，25（1）：90-102.
10	CHAI F， GAN L， YU Y J ．Magnetic field analysis of an iron-cored tiered type permanent magnet spherical motor using modified dynamic reluctance mesh method ［J］．IEEE Transactions on Industrial Electronics，2020，67（8）：6742-6751.
11	GAN L， PEI Y L， CHAI F ．Tilting torque calculation of a novel tiered type permanent magnet spherical motor ［J］．IEEE Transactions on Industrial Electronics，2020，67（1）：421-431.
12	CHAI F， GAN L， CHEN L ．A novel tiered type permanent magnet spherical motor and its rotor orientation measurement principle［J］．IEEE Access，2020（8）：15303-15312.
13	CHO S， LIM J S， OH Y J，et al ．A study on output characteristics of the spherical multi-dof motor according to the number of phases and pole pitch angles［J］．IEEE Transactions on Magnetics，2018，54（11）：1-5.
14	LI Zh， YU X Z， WANG X T，et al ．Cogging torque analysis of a special multi-dof permanent magnet motor ［C］∥Proceedings of IEEE International Conference on Applied Superconductivity and Electromagnetic Devices （ASEMD）．Tianjin：IEEE： 1-2.
15	XU L， ZHAO W X， JI J H，et al ．Design and analysis of a new linear hybrid excited flux reversal motor with inset permanent magnets［J］．IEEE Transactions on Magnetics，2014，50（11）：1-4.
16	郭凯凯，郭有光．磁通反向直线旋转永磁电机三维非线性等效磁路模型分析［J］．电工技术学报，2020，35（20）：4278-4286.
	GUO Kaikai， GUO Youguang ．3D nonlinear equivalent magnetic circuit model analysis of a flux reversal linear rotary permanent magnet machine［J］．Transactions of China Electrotechnical Society，2020，35（20）：4278-4286.
17	DRISCOLL T A， TREFETHEN L N ．Schwarz-christoffel mapping［M］．Cambridge：Cambridge University Press，2002.
18	DRISCOLL T A ．Schwarz-Christoffel toolbox user’s guide：version 2.3［M］．Newark：Department of Mathematical Sciences，University of Delaware，2005.
19	HAGUE B ．Electromagnetic problems in electrical engineering［M］．London：Oxford University Press，1929.
20	ROY D， SENGUPTA M ．An experimentally validated novel analytical approach for modeling of a saturated switched reluctance motor［J］．IEEE Transactions on Magnetics，2022，58（12）：1-11.
21	KIMIABEIGI M， WIDMER J D， LONG R，et al ．High-performance low-cost electric motor for electric vehicles using ferrite magnets［J］．IEEE Transactions on Industrial Electronics，2016，63（1）：113-122.
22	李争，何威，刘令旗，等．混合驱动式永磁转子三自由度电机的磁场分析和转矩计算［J］．电工技术学报，2018，33（s1）：49-57.
	LI Zheng， HE Wei， LIU Lingqi，et al ．Magnetic field analysis and torque calculation of hybrid drive type permanent magnet three-degree-of-freedom motor［J］．Transactions of China Electrotechnical Society，2018，33（s1）：49-57.
23	CHAI F， GAN L， PEI Y L，et al ．Tilting torque analysis of an iron-cored tiered-type permanent magnet spherical motor［J］．IEEE Transactions on Industrial Electronics，2021，68（7）：6121-6130.

参数	数值
定子外径/mm	166
定子内径/mm	53
气隙长度/mm	0.7
转子整体高度/mm	76
定子铁心单元数	12
转子永磁体极数	10
转子凸极齿距/（°）	9
偏转定子槽距/（°）	12

[1]	谢志辉, 刘瀚钰, 吴杰长, 等. 管壳式换热器热-磁-流耦合强化换热数值分析[J]. 华南理工大学学报(自然科学版), 2023, 51(3): 22-32.
[2]	张富臣, 李红梅, 赵春田, 等. 应力/激励磁场与力磁效应关联关系研究[J]. 华南理工大学学报(自然科学版), 2022, 50(3): 133-139.
[3]	陈志坚, 王雨晨, 黄鹏程, 等. 应用于近场测量的超宽带有源磁场探头设计[J]. 华南理工大学学报（自然科学版）, 2021, 49(6): 131-140.
[4]	罗玉涛, 周天阳, 许晓通. 基于遗传算法的四轮转向－驱动汽车时变 LQR 控制[J]. 华南理工大学学报（自然科学版）, 2021, 49(3): 114-122.
[5]	侯亮, 赖伟群, 崔凯, 等. 轴向柱塞马达平面配流副油膜润滑特性建模及分析[J]. 华南理工大学学报(自然科学版), 2021, 49(2): 99-109,139.
[6]	尹华杰郭志宇曾君. 滑模无速度传感器感应电机的简化预测转矩控制 [J]. 华南理工大学学报(自然科学版), 2020, 48(12): 18-26.
[7]	熊会元何山查鸿山朱雄来. 双轴驱动纯电动汽车驱动转矩的分配控制策略[J]. 华南理工大学学报(自然科学版), 2018, 46(11): 117-124.
[8]	罗小平李海燕唐杨吴迪. 磁纳米流体对微细通道饱和沸腾 CHF 特性的影响[J]. 华南理工大学学报（自然科学版）, 2018, 46(1): 1-8.
[9]	王振宝秦四成. 装载机液力变矩器的动态特性分析[J]. 华南理工大学学报（自然科学版）, 2016, 44(7): 41-46.
[10]	汤何胜訚耀保李晶. 轴向柱塞泵滑靴副间隙泄漏及摩擦转矩特性[J]. 华南理工大学学报（自然科学版）, 2014, 42(7): 74-79.
[11]	闻德生周瑞彬吕建森陈功杜永帅杨小勇. 双转子凸轮马达的转矩和转速分析[J]. 华南理工大学学报（自然科学版）, 2014, 42(4): 105-110,117.
[12]	毛阳陈志勇史文库. 磁流变液双质量飞轮设计及性能研究[J]. 华南理工大学学报（自然科学版）, 2014, 42(11): 78-84.
[13]	贾润泽王义春周飞. 磁场作用下的磁流变液导电性和导热性[J]. 华南理工大学学报（自然科学版）, 2014, 42(10): 57-62.
[14]	陈星苑士华胡纪滨彭增雄魏宾. 采用分体式双转子电机的混合动力系统的控制[J]. 华南理工大学学报(自然科学版), 2012, 40(7): 95-100.
[15]	刘定平崔志鹏. 基于磁场强度的锅炉管内氧化皮测量精度实验[J]. 华南理工大学学报(自然科学版), 2012, 40(2): 136-141.