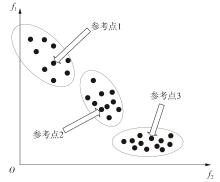
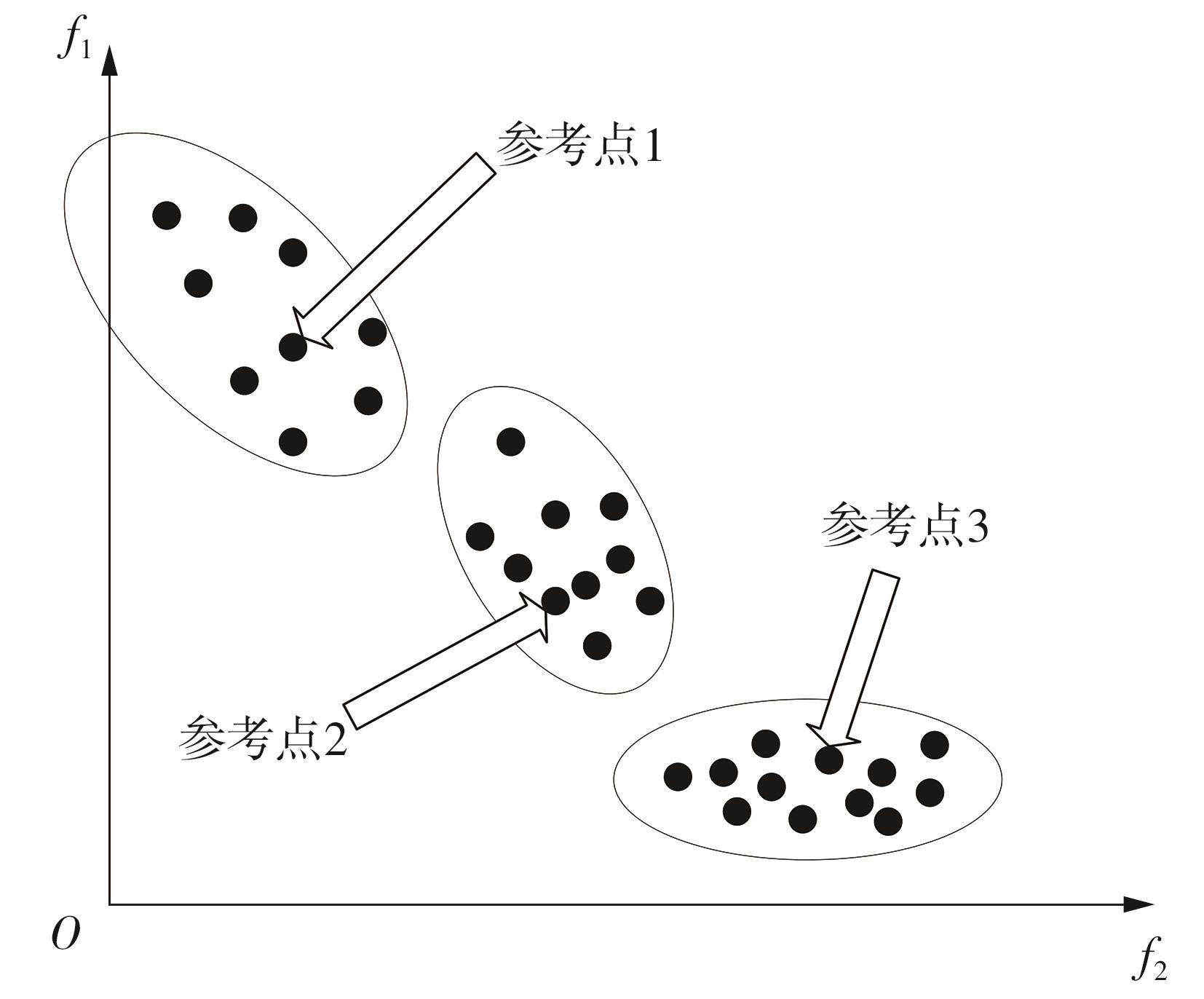
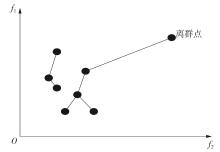
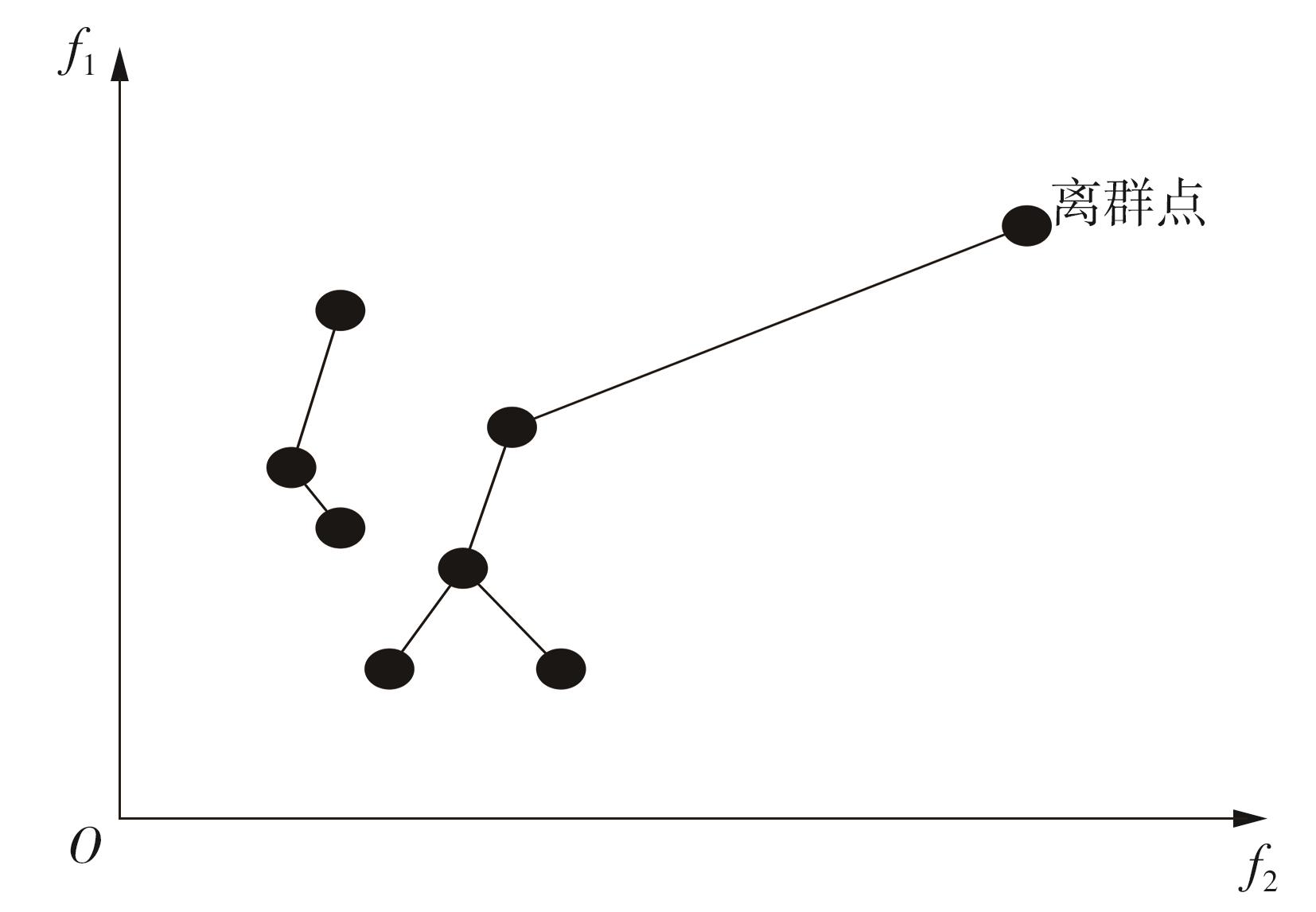
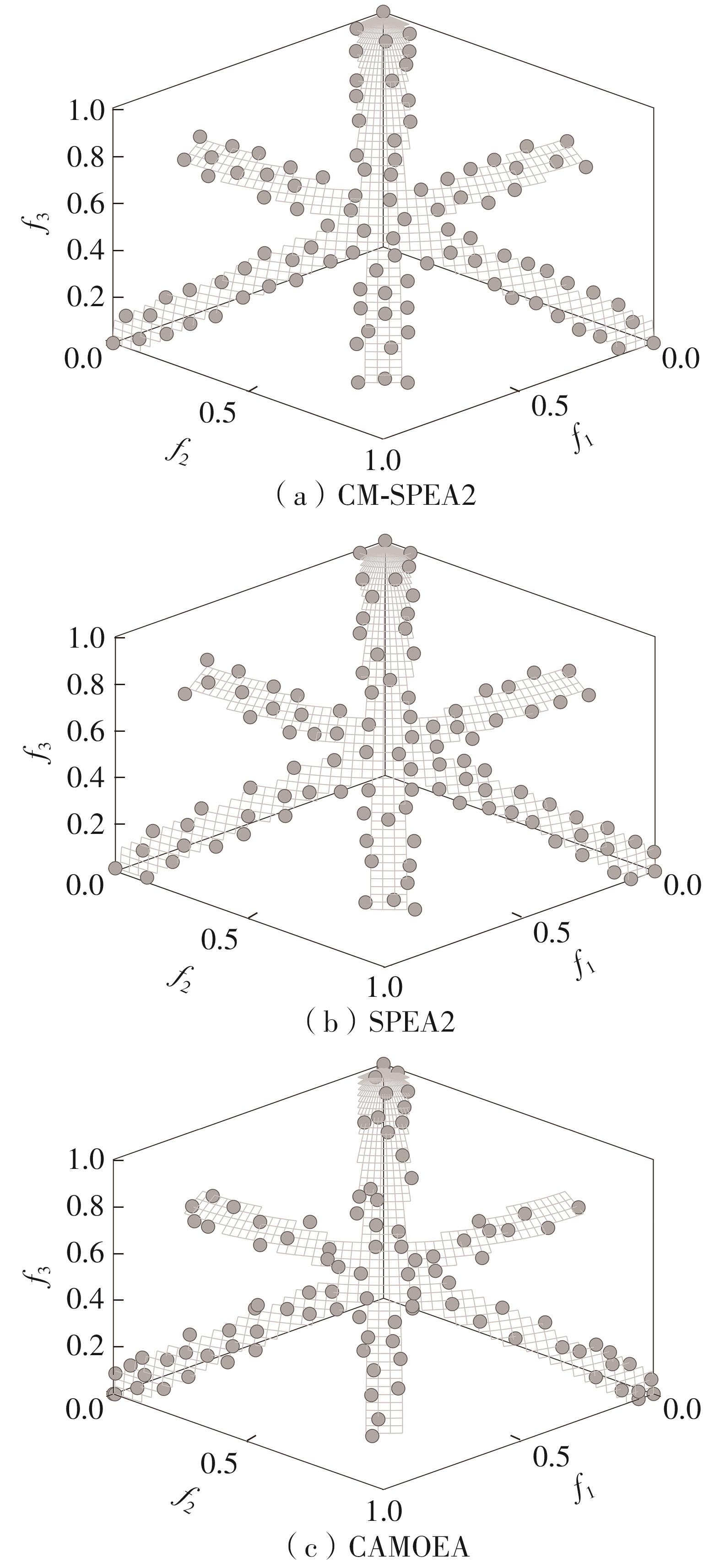
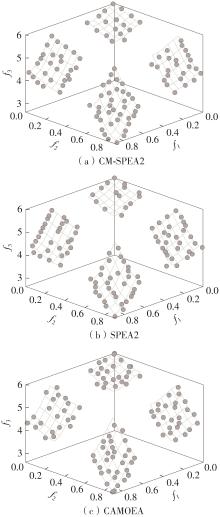
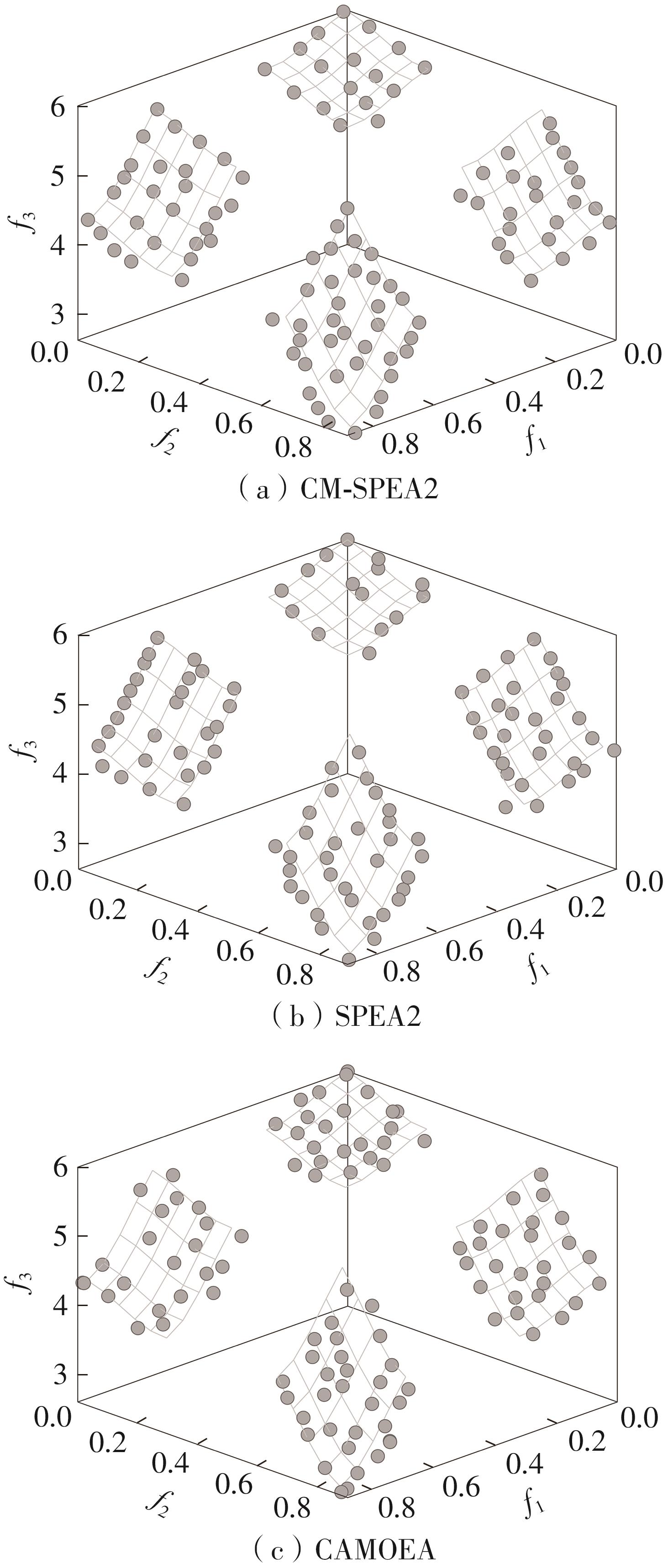
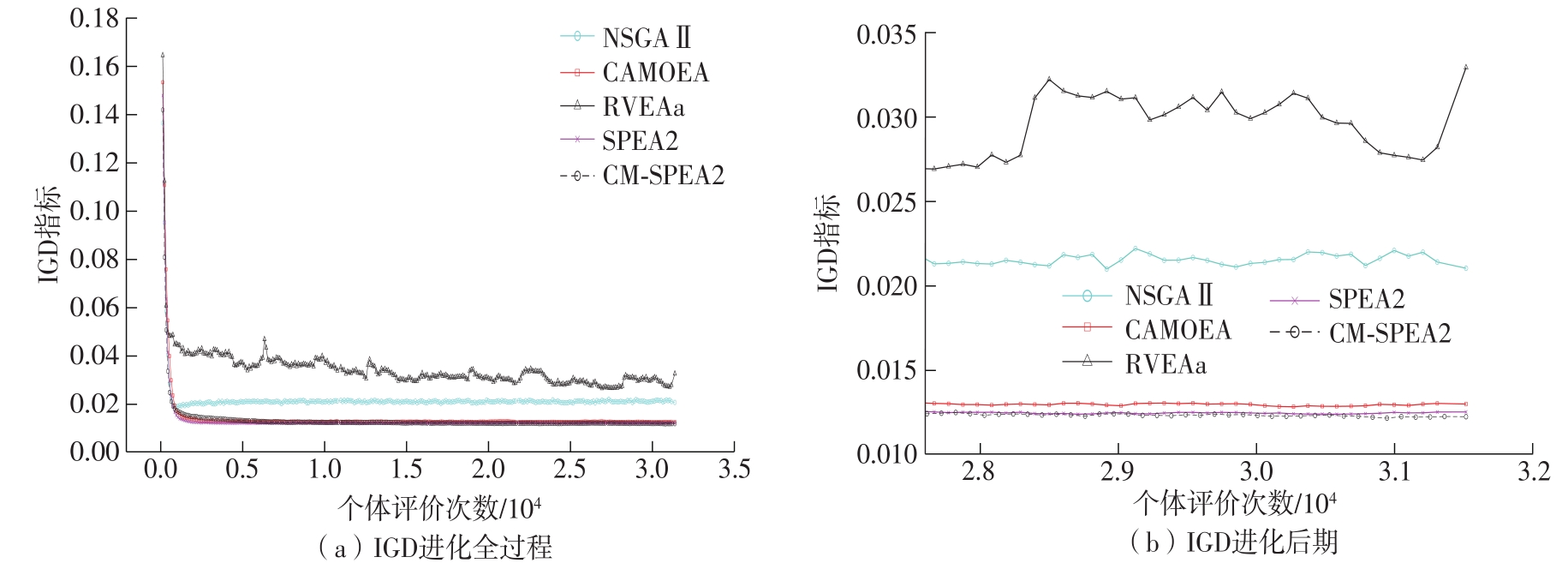
Journal of South China University of Technology(Natural Science Edition) ›› 2023, Vol. 51 ›› Issue (8): 137-148.doi: 10.12141/j.issn.1000-565X.220668
Special Issue: 2023年电子、通信与自动控制
• Electronics, Communication & Automation Technology • Previous Articles
Multi-Objective Optimization Based on Improved Distribution of Solutions
WANG Xuewu FANG Junyu GAO Jin GU Xingsheng
- Key Laboratory of Smart Manufacturing in Energy Chemical Process of the Ministry of Education,East China University of Science and Technology,Shanghai 200237,China
-
Received:2022-10-18Online:2023-08-25Published:2023-03-07 -
Contact:王学武(1972-),男,博士,副教授,主要从事智能优化算法、焊接机器人智能化技术、焊接自动化、系统建模、控制与优化研究。 E-mail:wangxuew@ecust.edu.cn -
About author:王学武(1972-),男,博士,副教授,主要从事智能优化算法、焊接机器人智能化技术、焊接自动化、系统建模、控制与优化研究。 -
Supported by:the National Natural Science Foundation of China(62076095)
CLC Number:
Cite this article
WANG Xuewu, FANG Junyu, GAO Jin, et al. Multi-Objective Optimization Based on Improved Distribution of Solutions[J]. Journal of South China University of Technology(Natural Science Edition), 2023, 51(8): 137-148.
share this article
Table 1
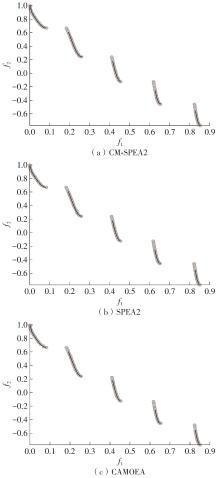
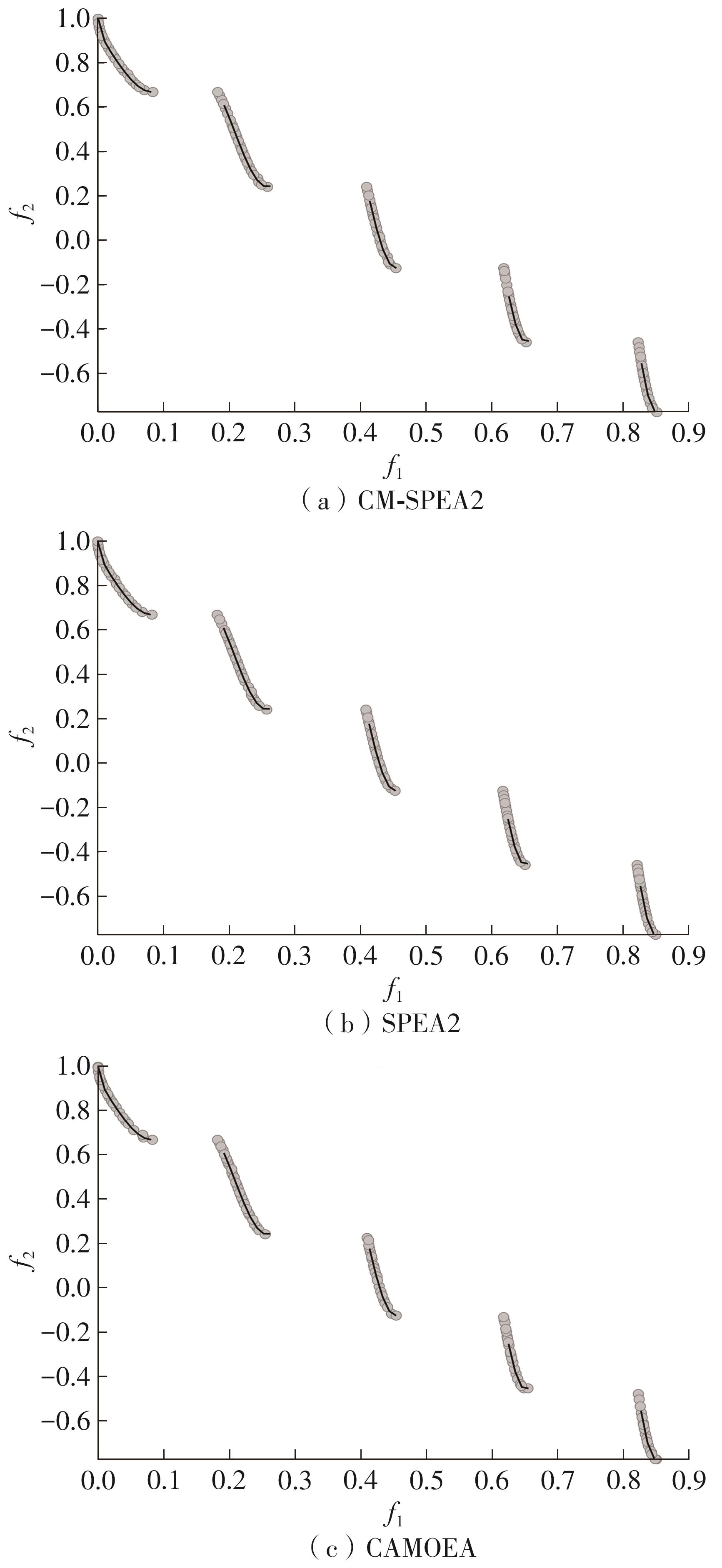
Comparison of IGD of different algorithms in multiple MOPs"
| 测试问题 | 目标数 | 数值类型 | 不同算法的IGD指标 | ||||
|---|---|---|---|---|---|---|---|
| CM-SPEA2 | SPEA2 | NSGAⅡ | CAMOEA | RVEAa | |||
| IMOP1 | 2 | 均值 标准差 | 6.374 5×10-3 1.02×10-3 | 5.109 4×10-3 3.14×10-4+ | 5.919 8×10-3 3.17×10-4= | 5.976 2×10-3 6.61×10-4= | 1.005 5×10-1 8.51×10-3‒ |
| IMOP2 | 2 | 均值 标准差 | 4.708 4×10-3 2.18×10-4 | 4.464 6×10-3 5.24×10-5+ | 5.177 6×10-3 2.56×10-4‒ | 5.177 6×10-3 2.56×10-4‒ | 5.698 0×10-3 2.98×10-3‒ |
| IMOP3 | 2 | 均值 标准差 | 3.365 8×10-3 3.77×10-5 | 3.504 2×10-3 5.06×10-5‒ | 3.993 2×10-3 1.07×10-4‒ | 3.659 2×10-3 8.54×10-5‒ | 2.753 7×10-2 5.20×10-2‒ |
| IMOP4 | 3 | 均值 标准差 | 6.677 1×10-3 7.41×10-5 | 6.945 9×10-3 1.63×10-4‒ | 8.665 5×10-3 4.65×10-4‒ | 7.618 3×10-3 2.01×10-4‒ | 1.392 5×10-2 2.07×10-3‒ |
| IMOP5 | 3 | 均值 标准差 | 3.245 7×10-2 4.41×10-4 | 3.286 0×10-2 5.09×10-4‒ | 4.620 5×10-2 2.97×10-3‒ | 3.391 9×10-2 8.73×10-4‒ | 3.370 4×10-2 1.09×10-3‒ |
| IMOP6 | 3 | 均值 标准差 | 1.163 8×10-1 1.97×10-1 | 1.165 5×10-1 1.97×10-1= | 5.082 1×10-2 5.27×10-3+ | 3.150 0×10-2 5.85×10-4+ | 9.782 5×10-2 1.56×10-1+ |
| IMOP7 | 3 | 均值 标准差 | 3.638 3×10-2 6.28×10-4 | 5.552 1×10-2 1.10×10-1‒ | 4.696 9×10-2 3.32×10-3‒ | 3.716 1×10-2 9.84×10-4‒ | 1.137 6×10-1 2.01×10-1= |
| IMOP8 | 3 | 均值 标准差 | 1.264 1×10-1 1.48×10-1 | 1.108 6×10-1 1.14×10-1+ | 1.069 4×10-1 5.24×10-3+ | 8.541 8×10-2 3.68×10-3+ | 1.528 0×10-1 1.73×10-1‒ |
| ZDT1 | 2 | 均值 标准差 | 3.626 7×10-3 3.63×10-5 | 3.789 8×10-3 6.41×10-5‒ | 4.544 8×10-3 1.39×10-4‒ | 4.268 9×10-3 9.29×10-5‒ | 4.152 7×10-3 1.64×10-4‒ |
| ZDT2 | 2 | 均值 标准差 | 3.724 6×10-3 3.40×10-5 | 3.741 2×10-3 4.25×10-5= | 4.601 9×10-3 1.36×10-4‒ | 4.216 3×10-3 1.01×10-4‒ | 3.866 5×10-1 2.96×10-1‒ |
| ZDT3 | 2 | 均值 标准差 | 4.392 0×10-3 6.14×10-5 | 5.641 3×10-3 5.36×10-3‒ | 6.121 1×10-3 5.34×10-3‒ | 5.840 0×10-3 5.37×10-3‒ | 8.335 1×10-3 7.52×10-3‒ |
| ZDT4 | 2 | 均值 标准差 | 5.219 3×10-3 2.03×10-3 | 4.429 5×10-3 2.75×10-4= | 5.503 7×10-3 1.05×10-3‒ | 4.923 6×10-3 7.29×10-4= | 7.398 6×10-3 6.52×10-3‒ |
| ZDT6 | 2 | 均值 标准差 | 2.944 2×10-3 5.44×10-5 | 3.006 2×10-3 8.76×10-5‒ | 3.520 6×10-3 1.13×10-4‒ | 3.352 8×10-3 9.36×10-5‒ | 3.100 8×10-3 1.32×10-4‒ |
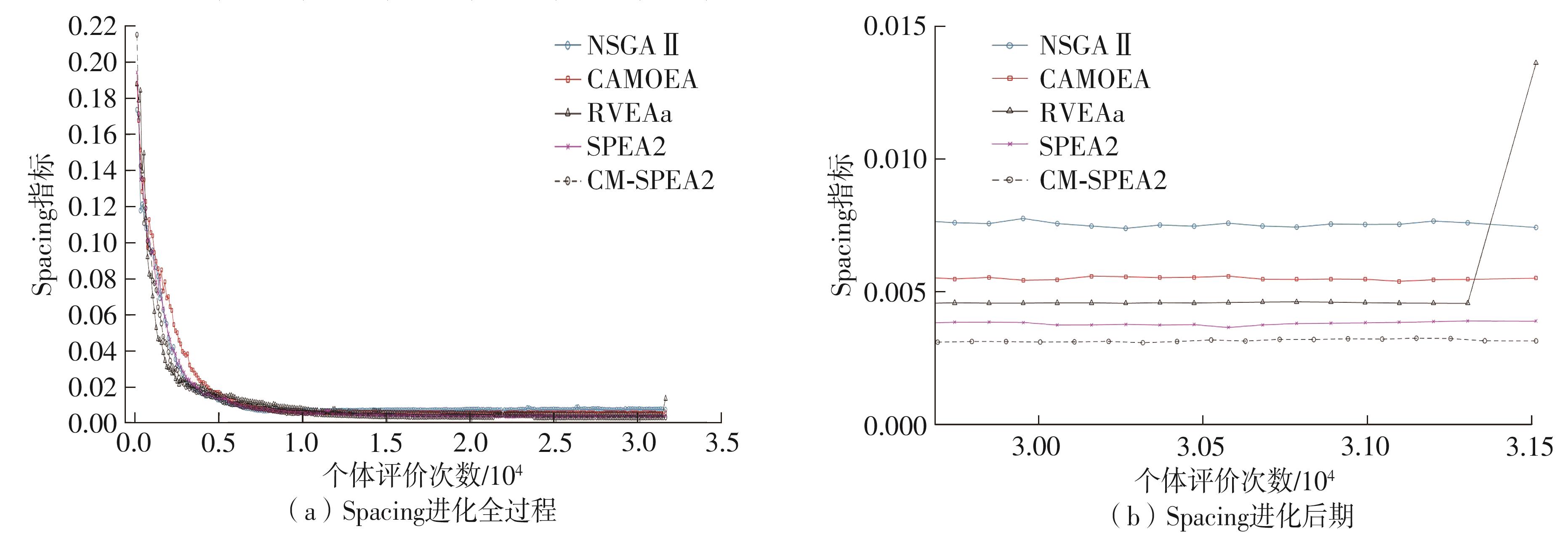
Table 2
Comparison of Spacing of different algorithms in multiple MOPs"
| 测试问题 | 目标数 | 数值类型 | 不同算法的IGD指标 | ||||
|---|---|---|---|---|---|---|---|
| CM-SPEA2 | SPEA2 | NSGAⅡ | CAMOEA | RVEAa | |||
| VNT1 | 3 | 均值 标准差 | 1.213 4×10-1 2.23×10-3 | 1.238 2×10-1 2.60×10-3‒ | 1.527 6×10-1 5.99×10-3‒ | 1.296 9×10-1 3.64×10-3‒ | 1.376 0×10-1 4.79×10-3‒ |
| VNT2 | 3 | 均值 标准差 | 1.214 3×10-2 4.11×10-4 | 1.240 3×10-2 4.15×10-4‒ | 2.141 7×10-2 1.62×10-3‒ | 1.277 5×10-2 4.24×10-4‒ | 3.111 1×10-2 7.72×10-3‒ |
| VNT3 | 3 | 均值 标准差 | 2.908 8×10-2 1.44×10-3 | 3.072 5×10-2 1.73×10-3‒ | 3.965 1×10-2 1.88×10-3‒ | 3.298 0×10-2 1.37×10-3‒ | 2.793 3×10-1 5.50×10-1‒ |
| +/‒/=数值 | 3/10/3 | 2/13/1 | 2/12/2 | 1/14/1 | +/‒/=数值 | ||
Table 2
Comparison of Spacing of different algorithms in multiple MOPs"
| 测试问题 | 目标数 | 数值类型 | 不同算法的Spacing指标 | |||||
|---|---|---|---|---|---|---|---|---|
| CM-SPEA2 | SPEA2 | NSGAⅡ | CAMOEA | RVEAa | ||||
| IMOP1 | 2 | 均值 标准差 | 3.274 1×10-3 5.97×10-4 | 3.321 5×10-3 2.87×10-4= | 6.458 5×10-3 5.30×10-4‒ | 4.857 6×10-3 6.15×10-4‒ | 5.087 2×10-2 7.18×10-3‒ | |
| IMOP2 | 2 | 均值 标准差 | 4.138 2×10-3 1.32×10-3 | 3.734 1×10-3 4.54×10-4= | 7.021 5×10-3 8.08×10-4‒ | 6.533 4×10-3 1.23×10-3‒ | 6.438 7×10-3 1.56×10-3‒ | |
| IMOP3 | 2 | 均值 标准差 | 2.252 1×10-3 2.36×10-4 | 2.813 2×10-3 3.36×10-4‒ | 5.647 3×10-3 5.21×10-4‒ | 4.419 5×10-3 4.32×10-4‒ | 1.109 2×10-2 1.80×10-3‒ | |
| IMOP4 | 3 | 均值 标准差 | 5.790 1×10-3 5.57×10-4 | 6.918 7×10-3 1.29×10-3‒ | 1.458 8×10-2 1.32×10-3‒ | 1.083 5×10-2 9.90×10-4‒ | 1.911 6×10-2 4.30×10-3‒ | |
| IMOP5 | 3 | 均值 标准差 | 1.359 8×10-2 1.53×10-3 | 1.535 8×10-2 1.66×10-3‒ | 4.005 5×10-2 3.95×10-3‒ | 3.014 1×10-2 2.05×10-3‒ | 2.997 1×10-2 3.02×10-3‒ | |
| IMOP6 | 3 | 均值 标准差 | 1.363 0×10-2 5.21×10-3 | 1.492 4×10-2 5.84×10-3‒ | 5.177 4×10-2 7.60×10-3‒ | 3.203 4×10-2 1.82×10-3‒ | 2.993 5×10-2 9.03×10-3‒ | |
| IMOP7 | 3 | 均值 标准差 | 1.701 3×10-2 2.26×10-3 | 1.808 3×10-2 3.82×10-3‒ | 4.246 9×10-2 6.53×10-3‒ | 2.968 4×10-2 2.39×10-3‒ | 2.227 5×10-2 8.51×10-3‒ | |
| IMOP8 | 3 | 均值 标准差 | 2.809 7×10-2 9.27×10-3 | 3.555 3×10-2 9.20×10-3‒ | 8.408 6×10-2 7.84×10-3‒ | 6.620 7×10-2 6.41×10-3‒ | 5.826 1×10-2 2.03×10-2‒ | |
| ZDT1 | 2 | 均值 标准差 | 2.636 3×10-3 2.74×10-4 | 3.121 9×10-3 3.12×10-4‒ | 6.549 2×10-3 5.36×10-4‒ | 5.160 0×10-3 3.90×10-4‒ | 1.252 0×10-2 1.43×10-3‒ | |
| ZDT2 | 2 | 均值 标准差 | 2.370 1×10-3 2.54×10-4 | 3.102 7×10-3 3.56×10-4‒ | 6.869 0×10-3 6.22×10-4‒ | 5.138 2×10-3 4.46×10-4‒ | 3.536 5×10-3 3.43×10-4‒ | |
| ZDT3 | 2 | 均值 标准差 | 3.170 5×10-3 4.16×10-4 | 3.808 2×10-3 4.32×10-4‒ | 7.464 9×10-3 8.15×10-4‒ | 5.560 7×10-3 5.16×10-4‒ | 1.329 0×10-2 1.36×10-3‒ | |
| ZDT4 | 2 | 均值 标准差 | 2.504 6×10-3 4.87×10-4 | 2.728 6×10-3 3.39×10-4‒ | 6.985 7×10-3 5.81×10-4‒ | 5.100 7×10-3 5.76×10-4‒ | 1.313 3×10-2 1.37×10-3‒ | |
| ZDT6 | 2 | 均值 标准差 | 1.891 8×10-3 1.80×10-4 | 5.604 1×10-3 1.85×10-2‒ | 5.706 7×10-3 5.17×10-4‒ | 4.158 5×10-3 3.99×10-4‒ | 2.312 7×10-3 2.28×10-4‒ | |
| VNT1 | 3 | 均值 标准差 | 5.834 5×10-2 9.33×10-3 | 6.365 0×10-2 5.72×10-3‒ | 1.383 4×10-1 1.47×10-2‒ | 1.082 0×10-1 7.05×10-3‒ | 1.216 5×10-1 1.88×10-2‒ | |
| VNT2 | 3 | 均值 标准差 | 8.735 3×10-3 1.12×10-3 | 7.623 8×10-3 8.57×10-4+ | 1.852 2×10-2 3.02×10-3‒ | 1.202 6×10-2 1.24×10-3‒ | 5.807 9×10-2 1.98×10-2‒ | |
| VNT3 | 3 | 均值 标准差 | 1.942 8×10-2 2.42×10-3 | 2.138 2×10-2 1.68×10-3‒ | 6.546 6×10-2 4.61×10-3‒ | 5.745 5×10-2 5.30×10-3‒ | 8.644 2×10-2 1.69×10-2‒ | |
| +/‒/=数值 | 1/13/2 | 0/16/0 | 0/16/0 | 0/16/0 | ||||
Table 3
Comparison of IGD and Spacing in multiple MOPs for CM-SPEA2 using different environment selection strategies"
| 测试问题 | 目标数 | IGD指标 | Spacing指标 | ||
|---|---|---|---|---|---|
| 策略1 | 策略2 | 策略1 | 策略2 | ||
| +/‒/=数值 | 1/2/13 | 2/9/5 | |||
| IMOP1 | 2 | 6.334 2×10-3 | 6.231 3×10-3= | 3.006 1×10-3 | 3.282 4×10-3‒ |
| IMOP2 | 2 | 4.896 0×10-3 | 4.727 7×10-3= | 4.905 4×10-3 | 4.828 8×10-1= |
| IMOP3 | 2 | 4.541 2×10-3 | 3.369 0×10-3+ | 2.215 8×10-3 | 2.227 5×10-3‒ |
| IMOP4 | 3 | 6.704 0×10-3 | 6.723 5×10-3= | 6.014 3×10-3 | 6.040 2×10-3= |
| IMOP5 | 3 | 3.244 5×10-2 | 3.254 3×10-2= | 1.370 7×10-2 | 1.385 1×10-2‒ |
| IMOP6 | 3 | 1.510 4×10-1 | 1.685 2×10-1= | 1.239 7×10-2 | 1.132 1×10-2= |
| IMOP7 | 3 | 5.648 7×10-2 | 7.650 2×10-2= | 1.600 7×10-2 | 1.603 0×10-2= |
| IMOP8 | 3 | 1.096 1×10-1 | 1.400 5×10-1= | 2.744 5×10-2 | 2.763 6×10-2‒ |
| ZDT1 | 2 | 3.622 9×10-3 | 3.631 1×10-3= | 2.589 1×10-3 | 2.561 9×10-3+ |
| ZDT2 | 2 | 3.731 9×10-3 | 3.729 2×10-3= | 2.451 7×10-3 | 2.500 3×10-3‒ |
| ZDT3 | 2 | 5.390 0×10-3 | 7.345 4×10-3‒ | 3.168 4×10-3 | 3.798 4×10-3 |
| ZDT4 | 2 | 4.644 3×10-3 | 4.834 7×10-3= | 2.403 1×10-3 | 2.520 9×10-3‒ |
| ZDT6 | 2 | 2.950 7×10-3 | 2.958 9×10-3= | 2.055 8×10-3 | 1.960 9×10-3= |
| VNT1 | 3 | 1.209 4×10-1 | 1.220 6×10-1‒ | 5.623 4×10-3 | 6.001 8×10-3‒ |
| VNT2 | 3 | 1.199 2×10-2 | 1.205 7×10-2= | 8.837 2×10-3 | 8.652 7×10-3+ |
| VNT3 | 3 | 2.941 6×10-2 | 2.916 4×10-2= | 1.927 2×10-2 | 1.902 9×10-2= |
| 1 | ZHANG Q, LI H .MOEA/D:a multiobjective evolutionary algorithm based on decomposition[J].IEEE Transactions on Evolutionary Computation,2007,11(6):712-731. |
| 2 | ZHANG C, GAO L, LI X,et al .Resetting weight vectors in MOEA/D for multiobjective optimization problems with discontinuous Pareto front[J].IEEE Transactions on Cybernetics,2022,52(9):9770-9783. |
| 3 | QI Y, LI X, YU J,et al .User-preference based decomposition in MOEA/D without using an ideal point[J].Swarm and Evolutionary Computation,2019,44:597-611. |
| 4 | DEB K, JAIN H .An evolutionary many-objective optimization algorithm using reference-point based non-dominated sorting approach,part I:solving problems with box constraints[J].IEEE Transactions on Evolutionary Computation,2014,18(4):577-601. |
| 5 | DENG W, ZHANG X, ZHOU Y,et al .An enhanced fast non-dominated solution sorting genetic algorithm for multi-objective problems[J].Information Sciences,2022,585:441-453. |
| 6 | TIAN Y, CHENG R, ZHANG X,et al .An indicator-based multiobjective evolutionary algorithm with reference point adaptation for better versatility[J].IEEE Transactions on Evolutionary Computation,2018,22(4):609-622. |
| 7 | TIAN Y, CHENG R, ZHANG X,et al .A strengthened dominance relation considering convergence and diversity for evolutionary many-objective optimization[J].IEEE Transactions on Evolutionary Computation,2019,23(2):331-345. |
| 8 | PANICHELLA A .An adaptive evolutionary algorithm based on non-Euclidean geometry for many-objective optimization[C]∥Proceedings of the Genetic and Evolutionary Computation Conference.New York:Association for Computing Machinery,2019:595-603. |
| 9 | SONODA T, NATAKA M .MOEA/D-S3:MOEA/D using SVM-based surrogates adjusted to subproblems for many objective optimization[C]∥Proceedings of the 2020 IEEE Congress on Evolutionary Computation (CEC).Glasgow:IEEE,2020:1-8. |
| 10 | ZITLER M, LAUMANNS M, THIELE L .SPEA2:improving the strength Pareto evolutionary algorithm[M].Berlin:Springer-Verlag,2002:95-100. |
| 11 | 翁理国,王安,夏旻,等 .基于局部搜索的改进SPEA2算法[J].计算机应用研究,2014,31(9):2617-2619,2639. |
| WENG Li-guo, WANG An, XIA Min,et al. Improved SPEA 2 based on local search[J].Application Research of Computers,2014,31(9):2617-2619,2639. | |
| 12 | 王学武,闵永,顾幸生 .基于密度聚类的多目标粒子群优化算法[J].华东理工大学学报(自然科学版),2019,45(3):449-457. |
| WANG Xuewu, MIN Yong, GU Xingsheng .Multi-objective particle swarm optimization algorithm based on density clustering[J].Journal of East China University of Science and Technology,2019,45(3):449-457. | |
| 13 | HUA Y, JIN Y, HAO K .A clustering-based adaptive evolutionary algorithm for multiobjective optimization with irregular Pareto fronts[J].IEEE Transactions on Cybernetics,2019(7):2758-2770. |
| 14 | 李密青,郑金华,李珂 .一种非均匀分布问题分布性维护方法[J].电子学报,2011,39(4):946-952. |
| LI Mi-qing, ZHENG Jin-hua, LI Ke .A diversity maintenance method for non-uniform distribution problem[J].Acta Electronica Sinica,2011,39(4):946-952. | |
| 15 | 郑金华,邹娟 .多目标进化优化[M].北京:科学出版社,2018:70-72. |
| 16 | TIAN Y, CHENG R, ZHANG X,et al .PlatEMO:a MATLAB platform for evolutionary multi-objective optimization[J].IEEE Computational Intelligence Magazine,2017,12(4):73-87. |
| 17 | WANG H, JIAO L, YAO X .Two_arch2:An improved two-archive algorithm for many-objective optimization[J].IEEE Transactions on Evolutionary Computation,2015,19(4):524-541. |
| 18 | COELLO C, CORTES N .Solving multiobjective optimization problems using an artificial immune system[J].Genetic Programming and Evolvable Machines,2005,6(2):163-190. |
| 19 | SCHOTT J .Fault tolerant design using single and multicriteria genetic algorithm optimization[D].Cambridge:Massachusetts Institute of Technology,1995:199-200. |
| 20 | 郭一楠,汤万宝,陈国玉,等 .动态多目标进化优化研究进展[J].信息与控制,2021,50(2):162-173. |
| GUO Yinan, TANG Wanbao, CHEN Guoyu,et al .Research progress on dynamic multi-objective evolutionary optimization[J].Information and Control,2021,50(2):162-173. |
| [1] | LI Shuxun, HU Yinggang, LI Cheng, et al. Optimization of Body Profile Line of Axial Flow Control Valve Based on Surrogate Model [J]. Journal of South China University of Technology(Natural Science Edition), 2023, 51(3): 41-52. |
| [2] | LIU Guoyong, ZHANG Wenpeng, ZHANG Tongxin, ZHU Dongmei, ZENG Xinxi. Structural Optimization and Flow Field Characteristics of Large Format SLM Forming Bin [J]. Journal of South China University of Technology(Natural Science Edition), 2023, 51(12): 53-63. |
| [3] | ZHAO Kegang, HE Kunyang, LI Jie, et al. Multi-objective Energy Management Strategy of HEV Based on Improved Dynamic Programming Method [J]. Journal of South China University of Technology(Natural Science Edition), 2022, 50(9): 138-148. |
| [4] | JIANG Tao, LU Zhou. Multi-objective Optimization of Flat Skylights in the Elevated Railway Station [J]. Journal of South China University of Technology(Natural Science Edition), 2022, 50(7): 13-24. |
| [5] | LIU Hanwu, LEI Yulong, FU Yao, et al. Adaptive Regenerative Braking Control Strategy of Range-Extended Electric Vehicle Based on Multi-Objective Optimization [J]. Journal of South China University of Technology (Natural Science Edition), 2021, 49(7): 42-50,65. |
| [6] | JIN Xia, HU Juncong, WANG Wei, et al. Adjustment Strategy for Automobile Hood Matching in Virtual Environment [J]. Journal of South China University of Technology (Natural Science Edition), 2020, 48(6): 87-96. |
| [7] | DAI Xiang HUANG Xifeng TANG Rui JIANG Mengting CHEN Xingshu WANG Haizhou LUO Liang. Subtopic Detection Algorithm Based on Hierarchical Clustering [J]. Journal of South China University of Technology (Natural Science Edition), 2019, 47(8): 84-95. |
| [8] |
WU Di LIU Li LI Xiaojun LIU Conghong.
Research on the Technologies of Passive Low Energy Buildings on the Basis of Multi-Objective Optimization Method———by Taking Cold Zone Residential Buildings for Example
|
| [9] | BAI Zhong-hao HE Cheng ZHU Feng. Analysis of Electromagnetic and Shock Wave Mitigation Capability of a Novel Sandwich Plate with Composites [J]. Journal of South China University of Technology (Natural Science Edition), 2016, 44(9): 137-143. |
| [10] | ZHAI Zhen-kun LI Di. Parameter Selection of CNC Real-Time Task on the Basis of Multi-Objective Optimization [J]. Journal of South China University of Technology (Natural Science Edition), 2016, 44(3): 23-28. |
| [11] | Zhou Jie Tao Ya-ping Zhang Jian-sheng Shao Chang-wei Luo Yan. Multi-Objective Optimization of Roll Forgings of Coupler Yoke Based on MBC Toolbox [J]. Journal of South China University of Technology (Natural Science Edition), 2015, 43(11): 61-66. |
| [12] | Yang Zhao-sheng Qu Xin Lin Ci-yun Bing Qi-chun Gong Bo-wen. Traffic Signal Optimization Method Considering Low Emissions and Short Delay [J]. Journal of South China University of Technology (Natural Science Edition), 2015, 43(10): 29-34,41. |
| [13] | Zhang Shu-qin Xia Hong-shan Jiang Yu Zhan Xu-ren. A Constrained Multi-Objective Genetic Simulated Annealing Algorithm for Runway Scheduling [J]. Journal of South China University of Technology (Natural Science Edition), 2015, 43(10): 35-41. |
| [14] | Wu Shan-yu Zhang Ping Li Fang. Multi-Task Scheduling Based on Particle Swarm Optimization in Cloud Manufacturing Systems [J]. Journal of South China University of Technology (Natural Science Edition), 2015, 43(1): 105-110. |
| [15] | Yu Li-min Xiong Hui-yuan Zong Zhi-jian Zhang Qing. Optimal Acceleration Control Strategy of Battery Electric Vehicles Based on Dynamic Programming [J]. Journal of South China University of Technology (Natural Science Edition), 2014, 42(8): 143-148. |
| Viewed | ||||||
|
Full text |
|
|||||
|
Abstract |
|
|||||