| 1 |
杨春玲,梁梓文 .特征域近端高维梯度下降图像压缩感知重构网络[J].华南理工大学学报(自然科学版),2024,52(3):119-130.
|
|
YANG Chunling, LIANG Ziwen .Feature-domain proximal high-dimensional gradient descent network for image compressed sensing[J].Journal of South China University of Technology (Natural Science Edition),2024,52(3):119-130.
|
| 2 |
TELLEZ D, LITJENS G, LAAK J V D,et al .Neural image compression for gigapixel histopathology image analysis[J].IEEE Transactions on Pattern Analysis and Machine Intelligence,2021,43(2):567-578.
|
| 3 |
YAN N, GAO C, LIU D,et al .SSSIC:semantics-to-signal scalable image coding with learned structural representations[J].IEEE Transactions on Image Processing,2021,30:8939-8954.
|
| 4 |
QIU H, ZHENG Q, MEMMI G,et al .Deep residual learning-based enhanced JPEG compression in the internet of things[J].IEEE Transactions on Industrial Informatics,2021,17(3):2124-2133.
|
| 5 |
SULLIVAN G J, TOPIWALA P N, LUTHRA A . The H.264/AVC advanced video coding standard:overview and introduction to the fidelity range extensions[C]∥ Proceedings of the SPIE 49th Annual Meeting on Optical Science and Technology.Denver:SPIE,2004:454-474.
|
| 6 |
WANG Yong, HUANG Jiwu .Robust AVS audio watermarking[J].Science China:Information Sciences,2010,53(3):607-618.
|
| 7 |
SRINIVASAN S, HSU P J, HOLCOMB T,et al .Windows media video 9:overview and applications[J].Signal Processing:Image Communication,2004,19(9):851-875.
|
| 8 |
CHEN Z, PAN X .An optimized rate control for low-delay H.265/HEVC[J].IEEE Transactions on Image Processing,2019,28(9):4541-4552.
|
| 9 |
ZHU B, LIU J Z, CAULEY S F,et al .Image reconstruction by domain-transform manifold learning[J].Nature,2018,555(7697):487-492.
|
| 10 |
CINTRA R J, BAYER F M, TABLADA C .Low-complexity 8-point DCT approximations based on integer functions[J].Signal Processing,2014,99:201-214.
|
| 11 |
TERRY-JACK M, O’KEEFE S .Fourier transform bounded Kolmogorov complexity[J].Physica D:Nonlinear Phenomena,2023,453:133824/1-7.
|
| 12 |
PARFIENIUK M .Lifting-based algorithms for computing the 4-point Walsh-Hadamard transform[C]∥ Proceedings of 2019 Signal Processing Symposium.Krakow:IEEE,2019:221-226.
|
| 13 |
SHAIK A, THANIKAISELVAN V .Comparative analysis of integer wavelet transforms in reversible data hiding using threshold based histogram modification [J].Journal of King Saud University:Computer and Information Sciences,2021,33(7):878-889.
|
| 14 |
LI Q, ELIE B Z, WILLIAM A .The impact and treatment of the Gibbs phenomenon in immersed boundary method simulations of momentum and scalar transport [J].Journal of Computational Physics,2016,310:237-251.
|
| 15 |
齐东旭,冯玉瑜 .关于Fourier—U级数的收敛性[J].中国科学技术大学学报,1983,13():7-17.
|
|
QI Dong-xu, FENG Yu-yu .On the convergence of Fourier-U series[J].Journal of China University of Science and Technology,1983,13(S1):7-17.
|
| 16 |
FENG Y, QI D .A sequence of piecewise orthogonal polynomials[J].SIAM Journal Mathematical Analysis,1984,15(4):834-844.
|
| 17 |
SONG R, MA H, WANG T,et al .The complete orthogonal V-system and its applications[J].Communications on Pure & Applied Analysis,2007,6(3):853-871.
|
| 18 |
WALSH J L .A closed set of normal orthogonal functions[J].American Journal of Mathematics,1923,45(1):5-24.
|
| 19 |
ALFRED H .Zur Theorie der orthogonalen Funktionensysteme[J].Mathematische Annalen,1910,69:331-371.
|
| 20 |
齐东旭,陶尘钧,宋瑞霞,等 .基于正交完备U-系统的参数曲线图组表达[J].计算机学报,2006,29(5):778-785.
|
|
QI Dong-xu, TAO Chen-jun, SONG Rui-xia,et al .Representation for a group of parametric curves based on the orthogonal complete U-system[J].Chinese Journal of Computers,2006,29(5):778-785.
|
| 21 |
YUAN X, CAI Z .A generalized Walsh system and its fast algorithm[J].IEEE Transactions on Signal Processing,2021,69:5222-5233.
|
| 22 |
齐东旭,宋瑞霞,李坚 .非连续正交函数[M].北京:科学出版社,2011.
|
| 23 |
ZHANG Y, CAI Z, XIONG G .A new image compression algorithm based on non-uniform partition and U-system[J].IEEE Transactions on Multimedia,2020,23:1069-1082.
|
| 24 |
ZHANG Y, CAI Z, YE B .An image importance partition-based compression method for COVID-19 computed tomography scan[J].IEEE Transactions on Industrial Informatics,2022,18(7):4596-4607.
|
| 25 |
熊刚强,齐东旭 .基于U-正交变换的图像编码算法[J].中国图象图形学报,2010,15(11):1569-1577.
|
|
XIONG Gangqiang, QI Dongxu .Algorithm of image encoding based on U-orthogonal transform[J].Journal of Image and Graphics,2010,15(11):1569-1577.
|
| 26 |
CHAM W K .Development of integer cosine transforms by the principle of dyadic symmetry[J].IEE Proceedings I:Communications,Speech and Vision,1989,136(4):276-282.
|
| 27 |
WANG Z.New algorithm for the slant transform[J].IEEE Transactions on Pattern Analysis and Machine Intelligence,1982,4(5):551-555.
|
| 28 |
YUAN X, CAI Z .ICHV:a new compression approach for industrial images[J].IEEE Transactions on Industrial Informatics,2021,18(7):4427-4435.
|
| 29 |
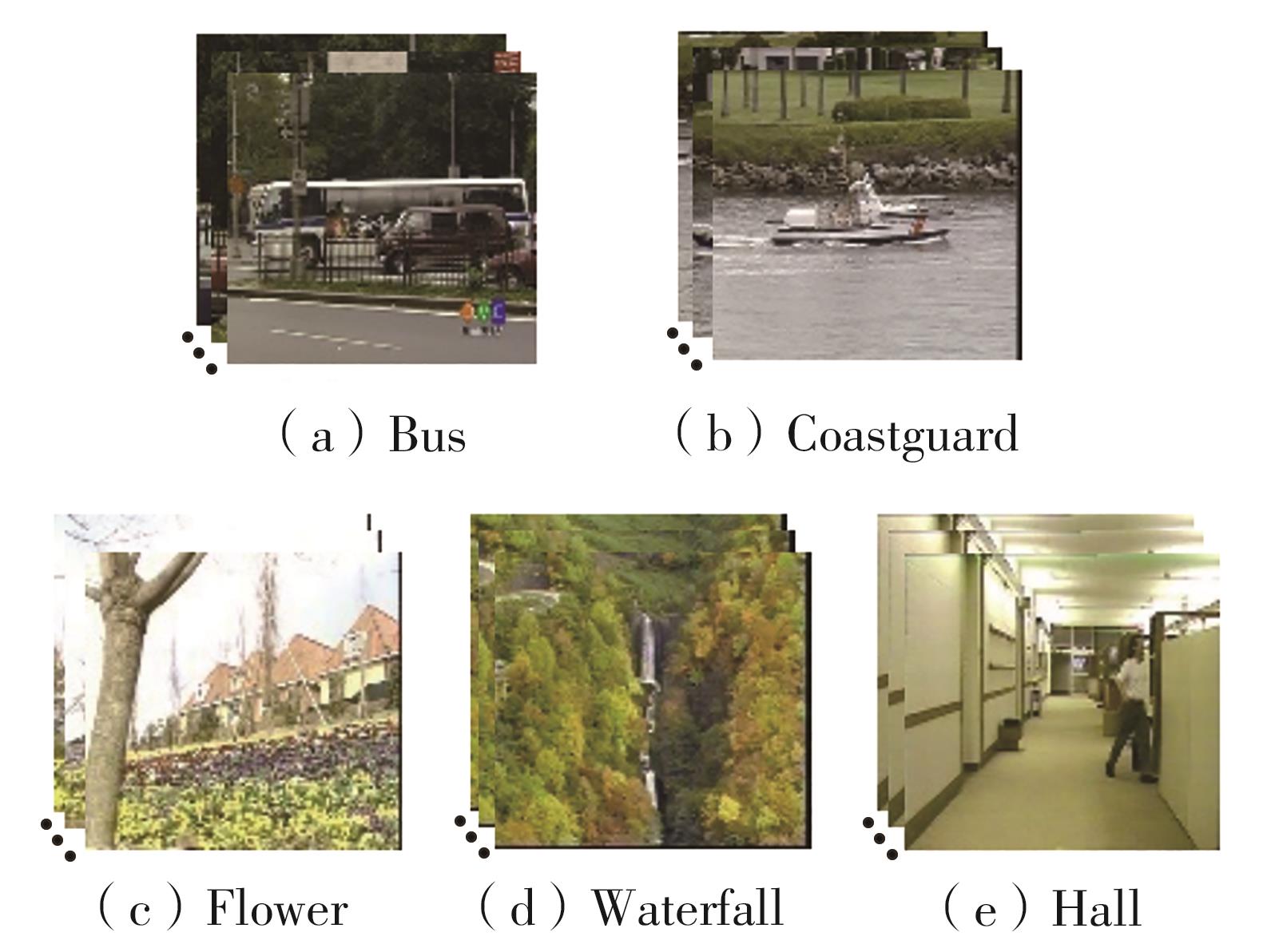
Computer Vision Group .Test images [EB/OL]. (2014-03-13)[2023-11-01]..
|
 ), CAI Zhanchuan3, SHI Wuzhen1(
), CAI Zhanchuan3, SHI Wuzhen1( ), YIN Wennan1
), YIN Wennan1